Nonlinear Systems/Differential Equations

Fixed Points

 

 

 

Def:         fixed point         value of state-variable vector where system is stationary

Tool:      To find a fixed point, set derivatives in autonomous form to zero, then solve for the state variables, x₁, ..., x_N, on the right-hand side.

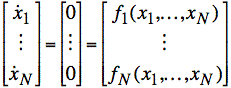           or    

Note:      If the solution of the system reaches a stable point, that stable point must be a fixed point.

Note:      The number of fixed points may be more or less than the number of equations.

Note:      A fixed point may be:

                  i)      stable (solutions converge toward them),

                  ii)     unstable (solutions diverge away from them),

iii)    saddle point (some state variables converge toward them, others diverge away from them).