Nonlinear Systems/Differential Equations

Linearization

Linear Approximation

 

 

Tool:      We linearize autonomous differential equations

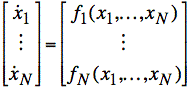               or        

                  using these steps:

i)      Defin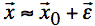e where .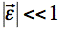

ii)     Expand the right-hand side in an N-dimensional Taylor series.

iii)    Discard terms of order 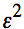or higher.

iv)    Observe that 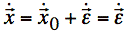since 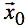is constant.

                  The result is an equation in terms of perturbation, 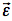, around a (constant) point, 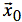, retaining only the constant (matrix) and linear (Jacobian) terms:

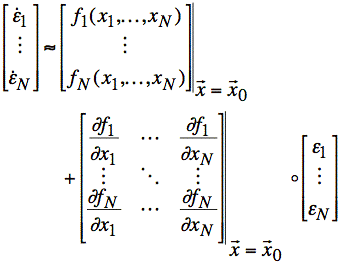
                  or

     

Note:      We usually linearize around in which case and the constant term disappears.