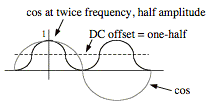
|
By: Neil
E. Cotter
|
Power electronics
|
|
|
AC
power
|
|
|
Tutorial
|
|
|
|
|
|
|
Tutorial: AC POWER
In
a linear circuit with sinusoidal source of frequency ω, currents and voltages are sinusoids of frequency ω. Power is still calculated as p = iv. Thus, we multiply sinusoids, although there is typically a phase
difference between the current and voltage. We define the phase difference as
(θv − θi).
If we also define im
and vm as the
amplitudes of current and voltage and adjust the origin of time so that θi = 0,
we have
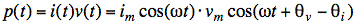
Note that we might try to leave the θi
in the current term but the result is quite awkward to work with.
We apply a standard trigonometric
identity to translate the product of sinusoids into a sum of sinusoids:
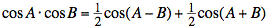
where A − B = θv − θi and
A + B = 2ωt + θv − θi.
The result is that the power has a
constant (or DC) term (that is no longer dependent on time or frequency)
and a sinusoidal signal (that has double the frequency of the current
and voltage):
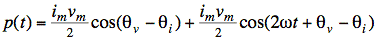
Note
also that there is a factor of one-half in both terms. A trick for remembering
these features of the power waveform is to consider the power waveform when
current and voltage are in phase. In that case, the product of i and v
has the shape of cos2(ωt).
Sketching cos2(ωt)
reveals that it is the sum of cos(2ωt) with amplitude one-half and a DC offset of
one-half.
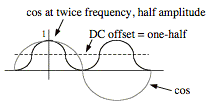
If we now think in terms of
frequency 2ω instead of ω we see that the second term of the power
expression is a cosinusoid with a magnitude and phase offset. In other words,
it is a sinusoidal signal represented in polar form. We may translate it into
rectangular form consisting of a pure cosine and a pure sine:
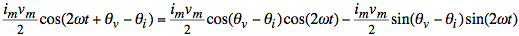
Note that this
is only the sinusoidal (or AC) part of the power expression.
To simplify the notation, we define P and Q:
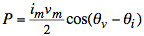
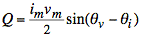
By coincidence, P appears twice in the complete power expression,
meaning we need only P and Q rather than three different terms:
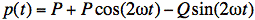
Because we have
both P and Q in the AC part of the power, (i.e., the last two
terms),we achieve an economy of notation (and possibly a loss of clarity) by
ignoring the DC part of the power and then using a phasor representation of the
AC part:
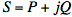
Note that the
sign is + for Q in the phasor, whereas
the sign is − for Q in the
expression for p. Also,
this "complex power", S,
happens to have, as its real part, the average or DC power P. Strictly speaking, however, the P represents the cosine part of the AC power.
If we use phasors for the original
current and voltage waveforms, we may derive the following identities:
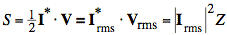
Once we have
found S, we know P and Q
and, hence, we know the complete power waveform, p(t).